Skip to main content

Measuring Impedance

 

Measuring Impedance

Filed in Basic Electronics on Dec.10, 2017

I saw a video today from one of the Youtube members that I subscribe to: Kevin Loughin KB9RLW.  In the video he was demonstrating how to use an oscilloscope and a signal generator to measure the impedance of a circuit ( in this case, it was simply a toroid coil).  The process made sense, but I was a little confused by his results.  He found that by decreasing the test frequency by half (10 Mhz down to 5 Mhz), his impedance went UP from 533 ohms to 2395 ohms!  I believe that is not reasonable, in that the impedance (inductive reactance, in this case) should have dropped by about half, to about 267 ohms.  Inductive reactance varies directly with frequency, not inversely, and not by 6 times with a simple halving of the test frequency.

So after checking all of the math, which seemed to be fine, I set up an experiment in my shop to check the results using the method Kevin was using.  Follow after the break to see my results…


 

MFJ Measurement of impedance of a T50-6 toroid with 12 turns

Kevin’s video is here:

 


I started by checking other sources for a similar procedure.  I found a source at http://www.zen22142.zen.co.uk/Theory/inzoz.htm

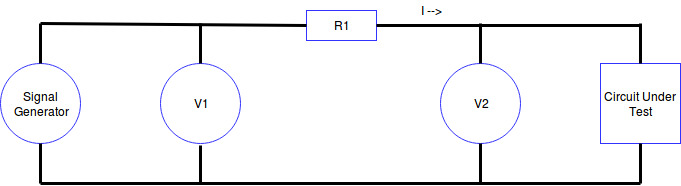
According to that source, the premise is that you can place a resistor that is in series with the rest of the circuit, then take AC voltage measurements on each side of the resistor (V1 & V2).  That allows you to calculate the current flow through the resistor (I).  Once you know the current flow in the circuit, you can use the voltage measurement on the circuit side of the resistor (V2) to calculate the impedance of the circuit.


 

Input Impedance Test

So first, find the current through the resistor:

Iin = V1 – V2 
    R1

Since the resistor and the circuit under test are in series, the current must be the same through both.  So Now use the V2 measurement and the current to determine the impedance of the circuit:

Z = V2 
Iin

The math appears to work out as I would expect it to.  So the basic procedure appears to be valid.

I built a spreadsheet and used Kevin’s measurements as input, and got the same results that he did.  But they still didn’t make sense.

So I set about duplicating the experiment here.  Since Kevin’s work pointed to a coil with 533 ohms of reactance at 10 mhz.

Let’s see, the formula for inductive reactance is Xl = 2*PI*F*L.  So dividing XL by (2*PI*F) should give us the inductance of the toroid.

L = 533/(2*PI*F)

L = 533/(6.28 * 10,000000)

L = 533/62,800,000

L = 0.000008487 henry, or 8.5 uh. I looked through my stash for something close.  And I found an 8 uh coil.

Next, I measured the reactance of the coil with my MFJ-259B antenna analyzer at 5 mhz.  I found the coil to measure 246 ohms at that frequency. That is pretty close to the calculated 240 ohms from the XL=2*PI*F*L formula.

 
Impedance at 5 Mhz

I also checked the reactance at 4 Mhz = 193 ohms, 3 Mhz = 142 ohms and 2 Mhz = 93 ohms, and found them all to be close to the calculated values for that inductor.

(Note that the impedance reading fluctuated a couple of counts, and I took an average of the reads as I read them, so the pictures may be off by a couple of counts)

 
Impedance at 4 Mhz

 

 
Impedance at 3 Mhz

 
Impedance at 2 Mhz

 

My MFJ analyzer could not read the reactance at 10 mhz for this coil, because it exceeded the 650 ohms limit of the analyzer.

So that confirmed for me that the impedance should go down as frequency goes down. Notice that the 2 Mhz reading is about half of the 4 Mhz reading.

Next, I built a jig to duplicate Kevin’s experiment.  I used a 1000 ohm resistor in series with the coil and took readings with my oscilloscope, duplicating Kevin’s test.

 
10 Mhz input voltage to resistor

 
10 Mhz Output voltage from resistor

 

  Coil measured Frequency Calculated Xl Measured Xl R Reference V1 V2 Z Calculated Error Percent
  7.65 uh 2 Mhz 96.132 93 1005 1.215 0.123 113.200 -17.75%
  7.65 uh 3 Mhz 144.199 142 1005 1.215 0.183 178.212 -23.59%
  7.65 uh 4 Mhz 192.265 193 1005 1.217 0.25 259.824 -35.14%
  7.65 uh 5 Mhz 240.331 246 1005 1.217 0.323 363.104 -51.08%
  7.65 uh 10 Mhz 480.663 N/A 1005 1.233 0.812 1938.384 -303.27%

You can see that the Z calculated starts off with a bit of an error at lower frequencies, and increases as the frequency is raised.  At 10 Mhz, the method is useless for this coil.  But in all cases, you can see that the impedance rises as frequency rises.

So why such a great error at higher frequencies?  I decided to continue the experiment, but using my Hewlett Packard 8640B signal generator as the source, rather than the antenna analyzer.  The results were nearly identical.  So it had nothing to do with the source.

Next, I thought that maybe the coil itself was flawed.  It’s possible that the capacitance between the windings, or core saturation, or something was contributing to the error.

I made a few more Xl calculations, and determined that a smaller coil of about 0.8 uh would result in measurements within the limits of my antenna analyzer.

So here is my new coil, wound on a T50-6 toroid core:

 
Smaller coil for testing

With this coil, the readings were more reasonable at the higher frequencies, good from 4 to 10 Mhz.


Coil Marked Coil measured Frequency Calculated Xl Measured Xl R Ref V1 V2 Z Calculated Error Percent
12t T50-6 0.727 uh 2 Mhz 9.135 9 1005 4.51 N/A    
12t T50-6 0.727 uh 3 Mhz 13.703 14 1005 4.48 0.042 9.511 30.59%
12t T50-6 0.727 uh 4 Mhz 18.271 18 1005 2.43 0.045 18.962 -3.78%
12t T50-6 0.727 uh 5 Mhz 22.839 23 1005 2.525 0.059 24.045 -5.28%
12t T50-6 0.727 uh 10 Mhz 45.67 46 1005 3.6 0.166 48.581 -6.36%
12t T50-6 0.727 uh 12 Mhz 54.814 55 1005 3.46 0.2 61.656 -12.48%
12t T50-6 0.727 uh 14 Mhz 63.950 65 1005 3.53 0.25 76.600 -19.78%
12t T50-6 0.727 uh 16 Mhz 73.086 76 1005 3.12 0.26 91.363 -25.01%
12t T50-6 0.727 uh 18 Mhz 82.221 86 1005 3.08 0.29 104.462 -27.05%
12t T50-6 0.727 uh 20 Mhz 91.357 96 1005 2.84 0.305 120.917 -32.36%

So what did I learn from all of this?  The method of using an oscilloscope and signal generator may be useful for finding the input impedance to a circuit or device, but try to make certain that you are testing at frequencies that are reasonable for the device.  For instance, it is probably not reasonable to use an 8 uh coil in a 10 Mhz circuit.  Stray capacitances, core saturation and maybe other effects may cause unexpected results.

Migrated Comments:

5 Responses to “Measuring Impedance”

  1. Lynn Says:
    January 1st, 2018 at 10:08 am

    Randy,

    That is a wonderful example of a well thought out experiment! It goes to prove what one of my old math instructors used to tell us and that is that you should have some idea of what the answer should be before you start solving the problem. Otherwise, how would you know if the answer you got was anywhere near what it should be. Also, once the answers you were getting still didn’t make all that much sense you went on to design a further experiment to investigate this. Very impressive!

    One must know the limitations of his instruments and tools before he can place a lot of faith in their results. Good job!

    73, Lynn, KJ3V

  2. wb0smx Says:
    January 1st, 2018 at 12:27 pm

    Thanks, Lynn! I learn more from failures than successes…

  3. Nor Amin Rohimi Says:
    August 27th, 2018 at 2:28 am

    I wonder Kevin if reading was influence by his system / component Parasitic Capacitance properties which lead to that high impedance at low freq.

  4. Kevin KB9RLW Says:
    May 7th, 2019 at 5:52 pm

    Howdy,

    Thank you for doing this. That quirky experiment has bugged me for a long time. There’s nothing like peer review to shake out results.

    That totally makes sense, exceeding the reasonable limits of the device causing the errors. I’ll comment this page on the video and pin it as I’m sure it will be useful to many.

    Kevin

  5. Kenneth Williams Says:
    June 9th, 2021 at 11:25 am

    Hi Randy

    Perhaps the measurements from KB8RLW are just fine.

    I also found this:
    https://ham.stackexchange.com/questions/13005/coax-or-bifilar-windings-for-a-choke and from the charts on this page, I also see impedance going up with lower frequency and peaking at a certain spot. Perhaps the coil that is being measured has sufficient inter-winding capacitance such that the capacitance becomes dominant as the frequency goes up and that his measurements were just fine for his specific coil and winding. I think that Nor Amin aluded to this with his comment.

Comments

Post a Comment

Popular posts from this blog

Building the W8NX Short Trap Dipole

Image
  Building the W8NX Short Trap Dipole Filed in Antennas on May.31, 2021 For the past five years, I have been operating the lower bands (30, 40 and 80 meters) with a trap dipole that was modeled after a web article written by John DeGood NU3E. [1] While the antenna performed well, a monsoon storm last summer beat it up enough that the antenna wire broke at the center balun. So I climbed the tower and lowered the remains of the antenna to the ground for repairs. On closer examination, I discovered that the relentless Arizona sun had taken its toll on the traps. The insulation on the exposed ends of the coax had rotted away. They were still working, but would need to be rebuilt and weatherized to insure good operation many years into the future. Since I was going to have to rebuild the antenna, I started thinking about whether this antenna met my current needs and wishes. I had been wanting to get on 160 meters at this QTH for some time, but my small back yard w...
Read more

Yaesu G-450XL Rotator Repair

Image
  Yaesu G-450XL Rotator Repair Filed in Antennas on Dec.30, 2007 In December of 2007, the rope support for the center element of my Cushcraft A3 broke, requiring me to climb the tower and bring the beam down for maintenance.  At the same time, at had been noticing that the rotator control was not indicating correctly, it was very erratic.  So I brought the rotator down for maintenance, as well. The first step was to mark the side of the case, where the top and bottom of the clam shells connected together.  This would allow me to assemble it in the correct alignment when the time came.   Marked Case of Rotator Next, I took the top cover off.  All of the bearings between the clam shells in the depression on the outside were safely stored.   Top Removed A little inspection revealed what the problem was.  The center gear had broken away from the shaft it was pressed onto.  I tried re-pressing it back onto the shaft, but it was to...
Read more

Kenwood TS-790A Light Replacement

Image
 I noticed a while back that my TS-790 meter displays had gone dim.  While I had the radio opened up to adjust the master clock. I decided to replace the burnt out incandescent lights with new white led lights. I neglected to take any pictures until after the work was done, but this will show where the lights are located, and which led bulbs I used to replace them. To access the 3 bulbs for replacement, I removed the top cover.  This and the bottom cover had already been removed in a prior repair in my case.  So I don't know if the bottom cover needs to come off or not. There are two screws on each side of the front faceplate.  I removed the top screw and loosened the bottom screw to allow the faceplate to tilt forward off the edge of the bench.   Faceplate Screws   Be careful when tipping the faceplate forward.  I managed to break one wire out of a connector on the bottom right side.  I was able to reattach the wire with the faceplate open....
Read more